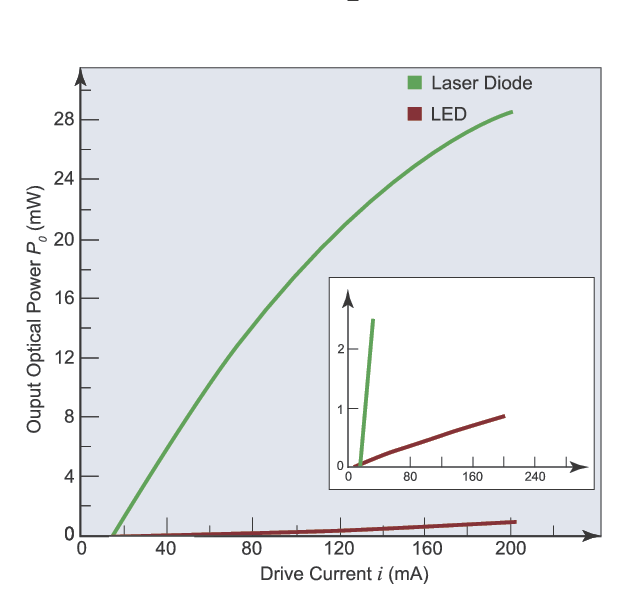
This relationship is observed in the form of a light-current (L-I) curve in Figure 2. The current value at which the line intersects the x-axis and produces zero emission is the threshold current for lasing (it). The slope of this curve above threshold is known as the differential responsivity (
Rd) and is typically expressed in units of W/A or mW/mA. This differential responsivity is determined by the external differential quantum efficiency (η
d), which essentially describes how efficient the laser system is in converting injected electrons into output photons. The efficiency is made up of an internal quantum efficiency (η
i), which is the ratio of generated photons (inside the junction) to injected electrons, and an extraction efficiency (ηe), which is associated with external losses (see section on extraction of light below). Equation (7) can be put in context with the generalized discussion of laser gain from
Critical Laser Components where gain was given as the product of the population difference (ΔN) and the transition cross section (σ). ΔN is essentially i divided by the volume of the junction (
V) while
ηi plays the role of the σ.
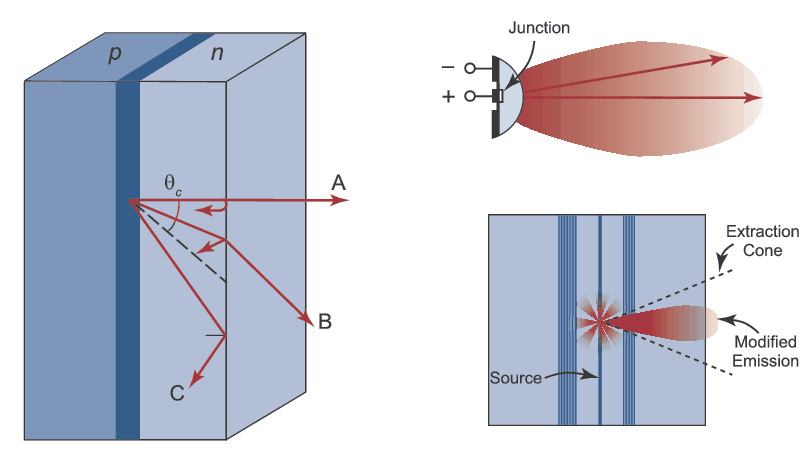
ηe accounts for the losses associated with the resonator cavity. Interestingly,
i/V can also be expressed as a current density
J divided by the thickness of the active region (l). In
Laser Diode and LED Physics, this reciprocal relationship between
J and
l was used to explain the benefit of moving from homojunction lasers to DH and QW devices.
 Over 8,000 products in-stock! & FREE 2-Day shipping on all web orders!* Learn More FREE T-Shirt with orders $250+ Details
Over 8,000 products in-stock! & FREE 2-Day shipping on all web orders!* Learn More FREE T-Shirt with orders $250+ Details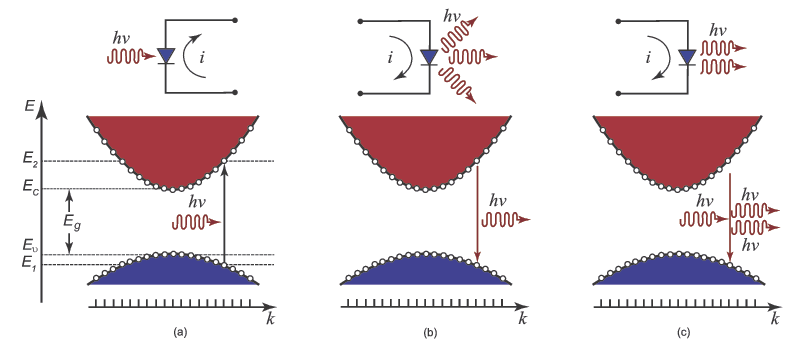
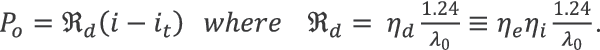


 Ultra-High Velocity
Ultra-High Velocity