Based on Light-Matter Interactions in Lasers, if N1 is greater than N2, absorption is the dominant process and the exponent in the above equation is negative, resulting in attenuation of the beam. In this case, the product of the population difference (ΔN) and the cross section (σ21) is known as the absorption coefficient (α), a measure of the loss in intensity per unit length. Conversely, if N2 exceeds N1, a population inversion exists and stimulated emission will dominate over absorption. The equation then possesses a positive exponent, giving rise to amplification. The product σ21ΔN is then defined as the gain coefficient (G) representing the gain per unit length. In order to maximize the amplification, a laser gain medium should possess a large transition cross section at the wavelength of interest and be able to support a significant population inversion.
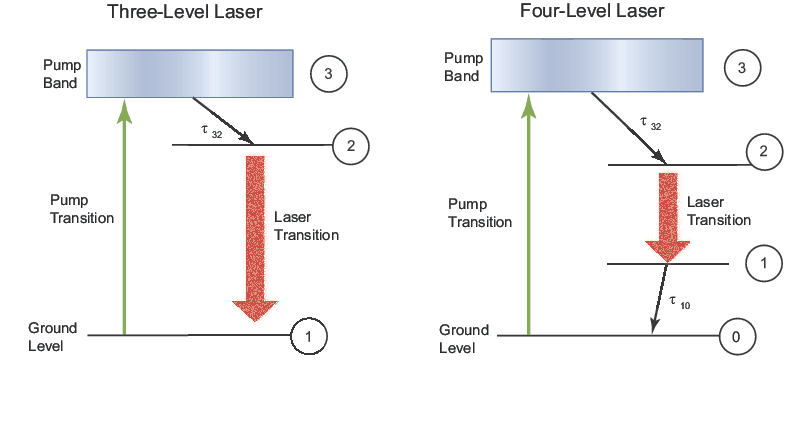
In practice, more than two energy levels are necessary to achieve a population inversion because a two-level scheme allows, at best, only equal populations of the upper and lower levels, thereby leading to no amplification. Alternatively, three- and four-level laser systems (as shown in Figure 2) essentially decouple the upper and lower levels. A three-level system pumps population from the ground state (level 1) into an excited state (level 3) which, rapidly decays to the level from which stimulated emission occurs (level 2). Level 2 is often referred to as the metastable level since it has a long lifetime. The lower level, where stimulated emission terminates (level 1), should be de-pumped rapidly to dispose of its population. These two requirements, i.e., long lifetime for upper level and short lifetime for lower level, help guarantee that a reasonably large population inversion can be maintained. Since level 1 is also the ground state, it possesses an inherently large population. Therefore, substantial pumping must be implemented to ensure that more than half of the atoms reach the metastable level 2. These disadvantages of a three-level system are avoided in a four-level system. Here, level 1 is situated above the ground state (level 0). Typically, rapid non-radiative decay depopulates this level, making a population inversion easier to achieve. State-level diagrams for a variety of laser media are given in Types of Lasers. Population densities also change dynamically depending on a variety of processes, including radiative and non-radiative decay pathways, pumping rates, as well as absorption and stimulated emission rates. Depictions of practical three- and four-level laser systems along with their decay pathways are shown in Figure 2.
 Over 8,000 products in-stock! & FREE 2-Day shipping on all web orders!* Learn More FREE T-Shirt with orders $250+ Details
Over 8,000 products in-stock! & FREE 2-Day shipping on all web orders!* Learn More FREE T-Shirt with orders $250+ Details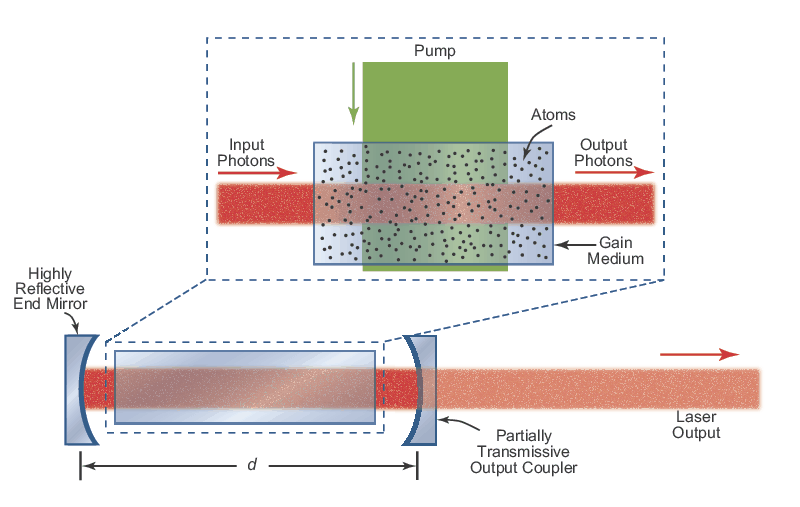
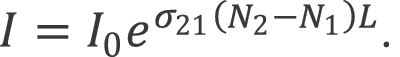
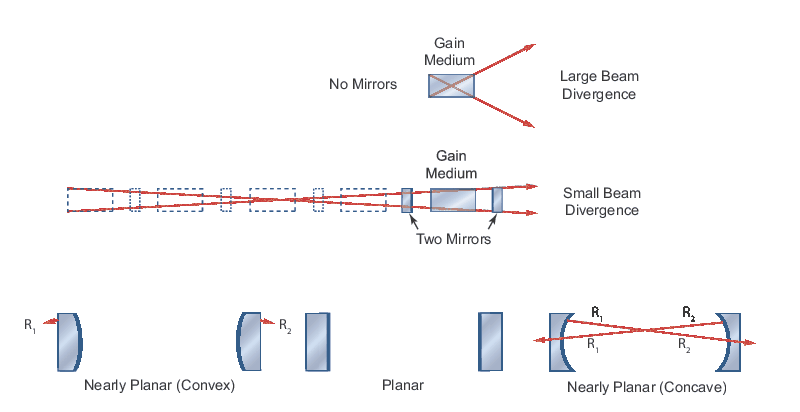
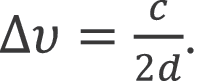
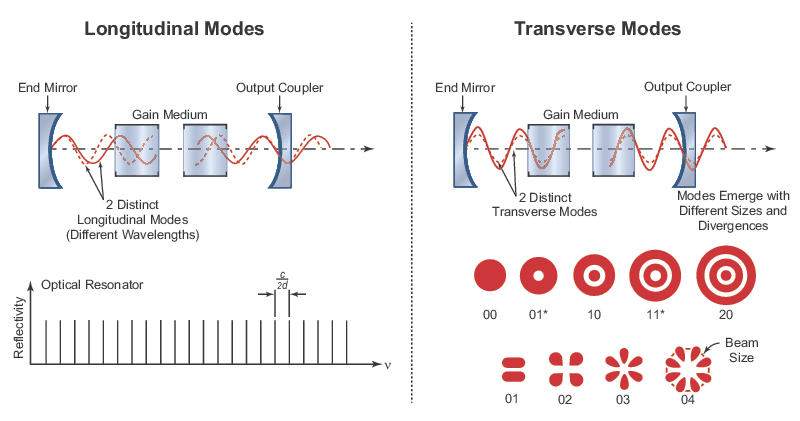

 Ultra-High Velocity
Ultra-High Velocity