When the source image in the slit plane is long then use the longest slits you can. Long slits give more throughput for a long source image on the slit. With the light from the model 6269 kW xenon lamp collected at F/1 and imaged at F/5, the arc image on the slit is about 16 mm tall. The 18 mm fixed slits of the 77200 give 30% more power through the 77200 than 12 mm slits on the Multiple Slit Wheel.
We referred above to the imaging of the input slit on the output. The useful slit length may be smaller than the 12 or 18 mm quoted if the output is to fall on a small sample, detector or fiber optic. In these cases, you must take care to concentrate the input flux on the area of the entrance slit that matches the useful zone of the exit slit. Longer slits do not necessarily give an advantage, and can add stray light to the system.
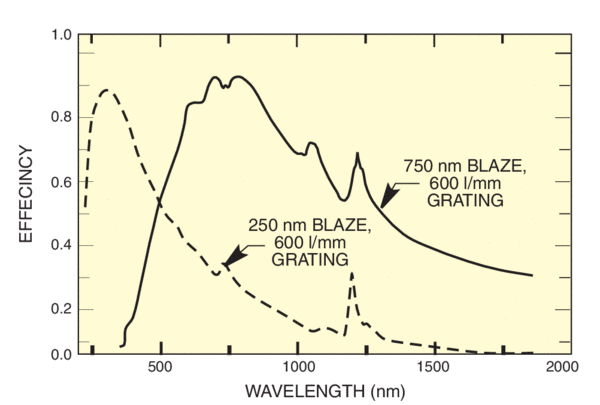
- Choose the most efficient grating
- Use the widest slits you can
- Use the longest slits practical
- Illuminate the input slit for most radiation in the useful zone of the output slit
Ensure that the long dimension of the source matches the long dimension of the slit. You may need to turn the monochromator on its side to achieve this.
The size of the primary radiating element is crucial to the choice of input optics. Element sizes may be found on see DC Arc Lamps for arc lamps and see Quartz Tungsten Halogen Lamps for QTH lamps. We deal summarily with the two extreme cases and at some length on the typical intermediate case.
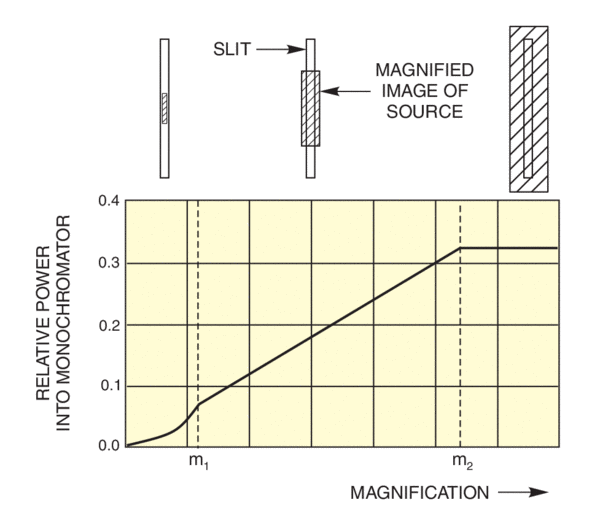
A large uniformly emitting source may fill the base of the acceptance cone. (Figure 2). Input optics will not increase the radiation through the monochromator, nor will moving the monochromator towards or away from the radiating area, providing the area continues to fill the base of the cone. To get most radiation through, use the largest slits you can. With a broadband source and a given bandwidth, the throughput of the 77200 1/4 m Monochromator can be 4.2 times that of the 77250 if you use the 18 mm long slits on the 77200.
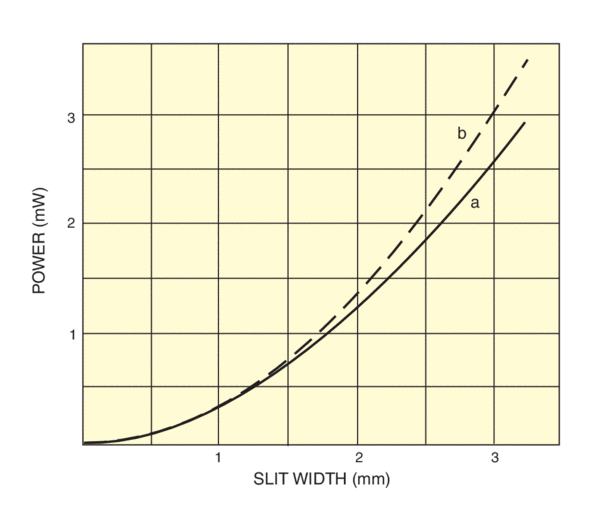
The theoretical point source does not exist, but some very small fiber optic sources, pinhole images and laser sources (or laser induced radiation) may approximate a point source of radiation. For the point source approximation, any magnified image of the radiating source should underfill the slit width.
With point sources, all you need to do is collect as much light as possible for the source using low F/# optics, and focus it on the slit opening at an F/number, which is equal to the monochromator F/number.
If you collect at F/2 from a 6 µm diameter single mode fiber and reimage on the slit at F/10, you might magnify by 5; the result (ignoring diffraction and lens aberrations) is about 30 µm image on the slit. If the slit width is 1.56 mm (5 nm bandpass with the 77700 and a 1200 l/mm grating), then the point source approximation is valid. Focusing at F/4 doesn’t increase the radiation through the monochromator, but it may give slightly better resolution since the input spot is smaller.
1. Any actual image size may be much larger than expected from a simple calculation because of optical aberrations and diffraction effects.
2. If you don't fill the input slit, the bandpass is not determined by the dispersion and slit sizes, but by a convolution of the input spot size and the output slit width.
3. Operating at high F/number leads to underfilling of the grating and improved resolution, but in extreme cases, loss of resolution (see Grating Physics).
4. Operating at high F/number with high power laser sources can lead to grating damage.
The source of radiation and slit size often have comparable dimensions. Our intense arc sources have dimensions from 0.25 x 0.25 mm, to 3.0 x 2.6 mm. The compact high brightness tungsten halogen filaments (see Quartz Tungsten Halogen Lamps) have dimensions from 1.7 x 0.65 to 6 x 16 mm. The slit dimensions for a 10 nm bandpass (with a 1200 l/mm grating) on the 77250 1/8 m Monochromator are 1.56 x 12 mm.
Fluorescing regions caused by focused beams from incoherent (i.e. non-laser) radiation also have mm dimensions.
We start by assuming that the monochromator, grating and slits are all optimized as previously suggested. We list two rules, comment extensively on them and then give two examples.
The two rules for most efficient coupling:
1. Always fill the monochromator acceptance cone.
2. Get as much light as possible through the slit while observing Rule 1.
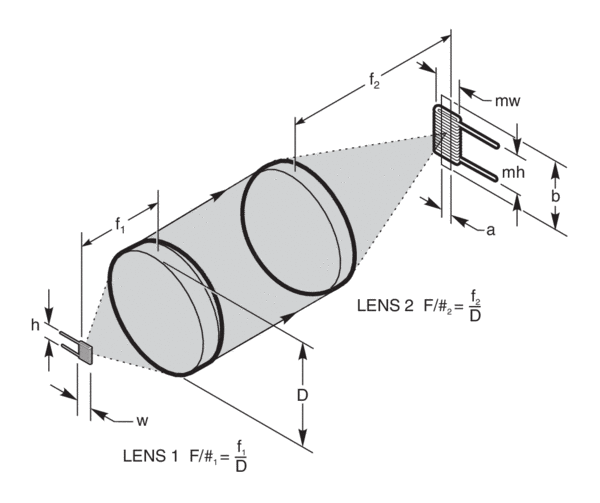
The problem is that the two rules are interlinked and can be satisfied by many optical systems. Most of our discussion deals with a two optic system; a condenser lens collecting and collimating light from the radiation source, and a focusing lens. Two lens systems, such as the one in Figure 5, simplify the design implementation because you can set the length of the collimated arm to suit your set up.
A single lens system is no better at satisfying our two rules, and you have the added complication of the relationships between:
- source-lens distance and light collection
- source-lens and lens-slit distances and source magnification
- lens-slit distance and focusing F/#
You also need to use a large diameter optic for efficient collection from the source. Our Monochromator Illuminators exemplify efficient implementation of a single optic solution.
PhotoMax™ Lamp Housing is designed for Rules 1 and 2. The ellipsoidal reflectors collect light very efficiently and focus it on the slit. If you use a low power arc lamp or other small volume radiating element, you will get more light into a monochromator using the appropriate PhotoMax™ than with any condenser system. (Note: it is crucial that the radiating system be small, ie. arc lamps with gaps less than approximately 1.5 mm.) Because selection of optics are not required, we will not discuss the PhotoMax™ systems in detail.
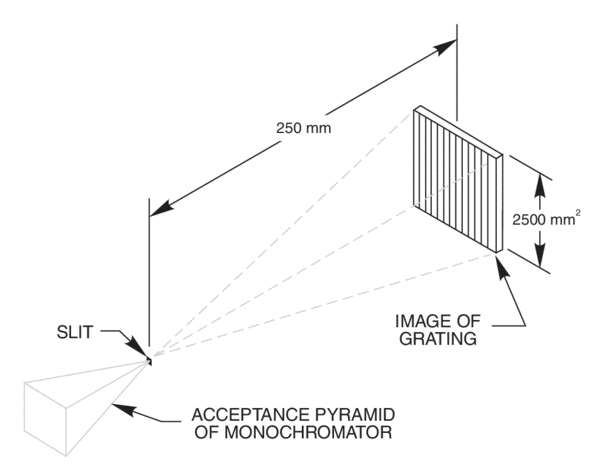
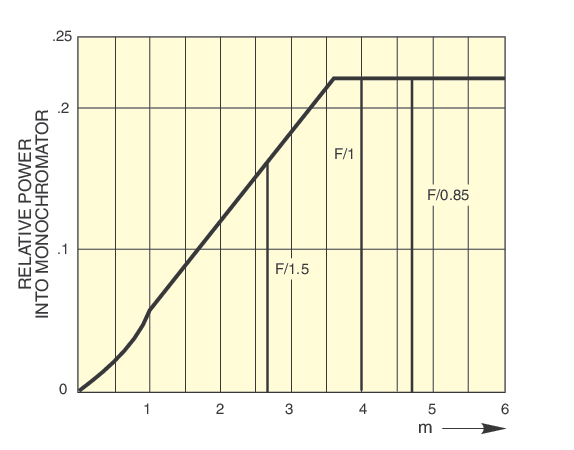
Filling the acceptance cone means focusing the beam on the slit at the correct F/number, ie. F/4 for the 77250, 77400 and 77700 or F/4.4 for the 77200. Focusing at these F/#s results in an overfill of the grating along vertical and horizontal diameters and underfill of the corners. If you focus at lower F/#, light is lost as it misses the grating and reflects inside the monochromator as stray light. All the light strikes the grating if you focus at a higher F/#, but the image of the radiating element on the slit is larger than it needs to be, with system efficiency loss.
Remember that the F/# quoted for monochromator input is the lens focal length divided by the beam diameter. This F/# is greater than the lens F/# since the beam usually does not completely fill the full lens aperture.
Figure 5 shows a typical optical system. Light from a filament is collected by Lens 1, and focused on the monochromator slit by Lens 2. The magnification, m, of the filament on the slit is given by the ratio of the F/numbers.
m = (F2/#)/(F1/#)
Because the beam is “collimated”, the beam diameter is approximately constant, so the magnification is also given by the ratio of the focal lengths, f2/f1.
Our condenser sources produce beams with diameters of 33 mm, 48 mm and 69 mm. Larger beam diameters require bulky and more expensive lenses. We use the 33 mm beams for lamps rated to 500 W and the 48 mm beams for lamps from 500 W - 1kW. Our Aspherabs® produce a 69 mm low aberration beam from any lamp.
Defining the beam diameter lets you fix Lens 2. For a 33 mm beam and the F/4.5 required for the 77200 Monochromator, Lens 2 should have a clear aperture of at least 33 mm and a focal length of 33 x 4.4 = 145.2 mm. Of the simple lens forms, plano-convex lenses give least aberration, so any 1.5 or 2 inch diameter plano-convex lens with 150 mm focal length can serve as Lens 2.
 Over 8,000 products in-stock! & FREE 2-Day shipping on all web orders!* Learn More FREE T-Shirt with orders $250+ Details
Over 8,000 products in-stock! & FREE 2-Day shipping on all web orders!* Learn More FREE T-Shirt with orders $250+ Details
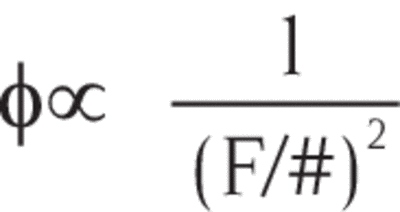
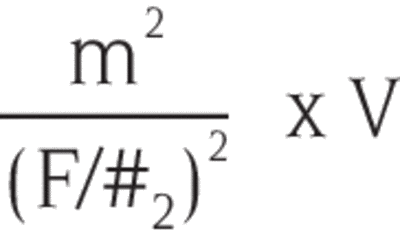
 Ultra-High Velocity
Ultra-High Velocity