In fact, it is at x = xR that R has its minimum value.
Note that these equations are also valid for negative values of x. We only imagined that the source of the beam was at x = 0; we could have created the same beam by creating a larger Gaussian beam with a negative wavefront curvature at some x < 0. This we can easily do with a lens, as shown in Figure 3.
The input to the lens is a Gaussian with diameter D and a wavefront radius of curvature which, when modified by the lens, will be R(x) given by the equation above with the lens located at -x from the beam waist at x = 0. That input Gaussian will also have a beam waist position and size associated with it. Thus we can generalize the law of propagation of a Gaussian through even a complicated optical system.
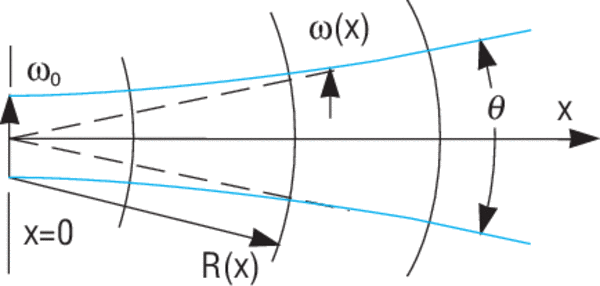
In the free space between lenses, mirrors and other optical elements, the position of the beam waist and the waist diameter completely describe the beam. When a beam passes through a lens, mirror, or dielectric interface, the wavefront curvature is changed, resulting in new values of waist position and waist diameter on the output side of the interface.
These equations, with input values for ω and R, allow the tracing of a Gaussian beam through any optical system with some restrictions: optical surfaces need to be spherical and with not-too-short focal lengths, so that beams do not change diameter too fast. These are exactly the analog of the paraxial restrictions used to simplify geometric optical propagation.
It turns out that we can put these laws in a form as convenient as the ABCD matrices used for geometric ray tracing. But there is a difference: ω(x) and R(x) do not transform in matrix fashion as r and u do for ray tracing; rather, they transform via a complex bi-linear transformation:
 Over 8,000 products in-stock! & FREE 2-Day shipping on all web orders!* Learn More FREE T-Shirt with orders $250+ Details
Over 8,000 products in-stock! & FREE 2-Day shipping on all web orders!* Learn More FREE T-Shirt with orders $250+ Details

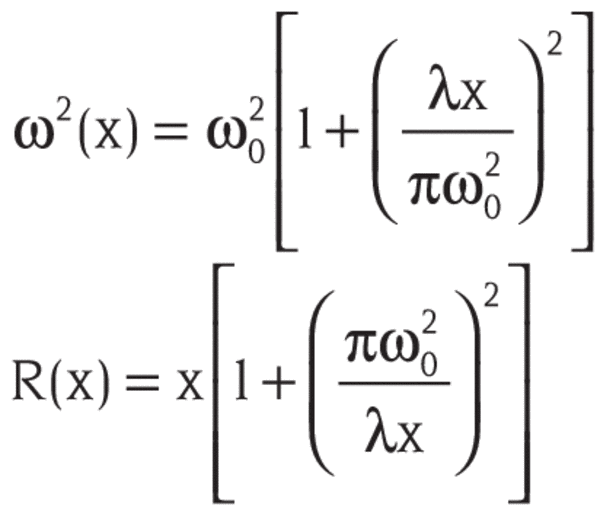
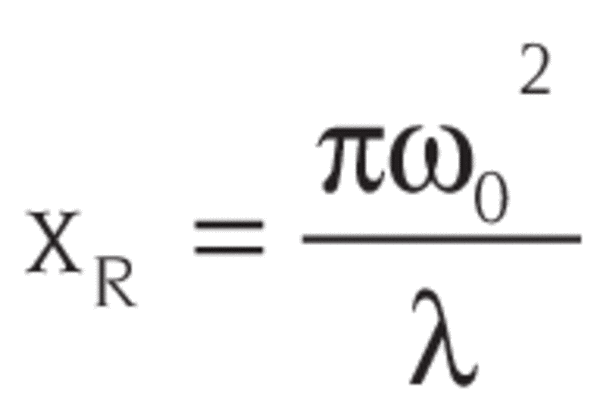
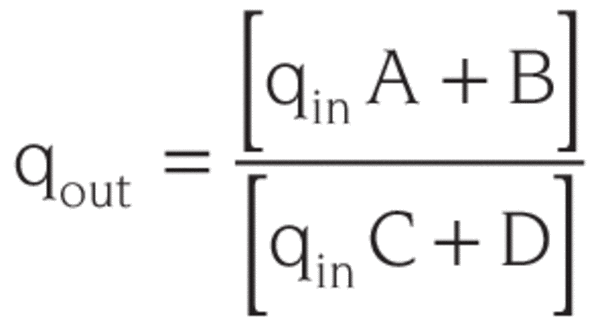
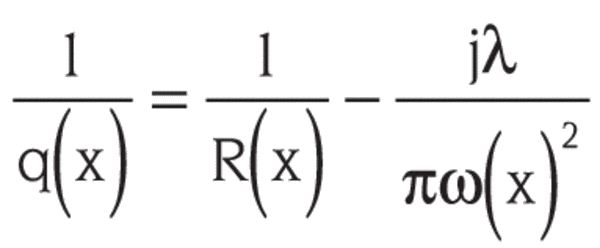
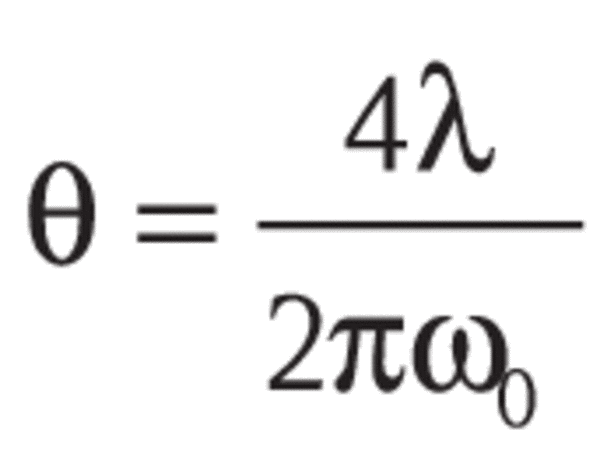
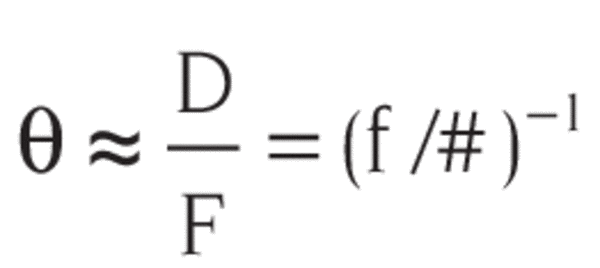
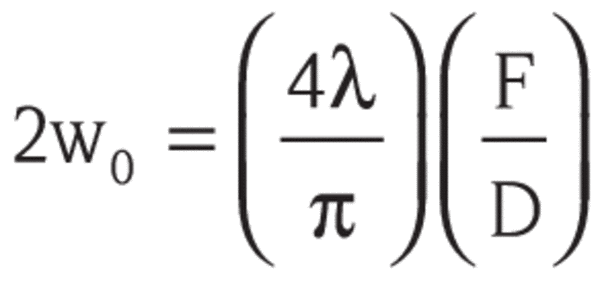
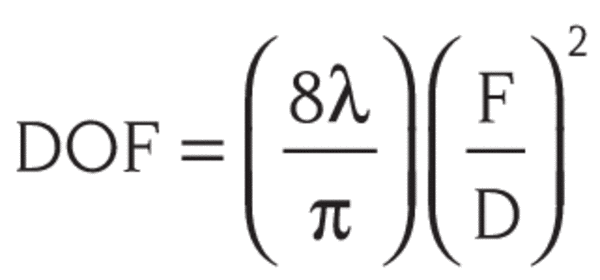
 Ultra-High Velocity
Ultra-High Velocity